Using the 5 Practices - Stacking Cups

If you’re interested in implementing 3 Act Math or other open-ended problems, check out 5 Practices for Orchestrating Productive Mathematics Discussions.

Learning Goal

“To ensure that a discussion will be productive, teachers need to have clear learning goals for what they are trying to accomplish in the lesson, and they must select a task that has the potential to help students achieve those goals…The key is to specify a goal that clearly identifies what students are to know and understand about mathematics as a result of their engagement in a particular lesson.” (Smith & Stein, 2011)

I had two learning goals for the lesson. (1)Provide a context for slope and y-intercepts. (2) Understand that linear functions have a constant rate of change and can be represented in multiple ways. I chose Stacking Cups because it’s my favorite activity for introducing linear functions. It lines up perfectly for slope-intercept form, and the kids always have fun stacking cups as tall as the teacher. I’m usually in favor of any hands-on component as well, and this lesson provides plenty of that.

Anticipating

“Anticipating students’ responses involves developing considered expectations about how students might mathematically interpret a problem, the array of strategies—both correct and incorrect—that they might use to tackle it, and how those strategies and interpretations might relate to the mathematical concepts, representations, procedures, and practices that the teacher would like his or her students to learn.” (Smith & Stein, 2011)

For the anticipation process, I tried to predict different strategies and mistakes by working the problem out in many ways. I created the following charts for organization.

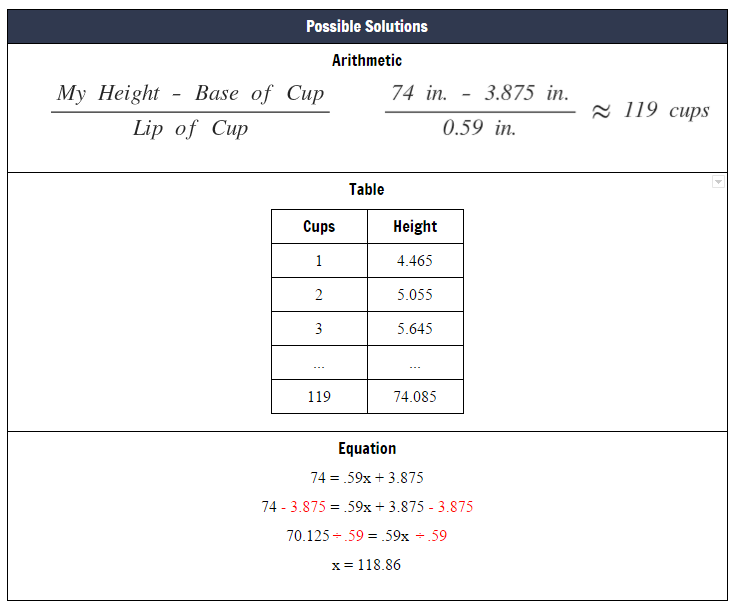
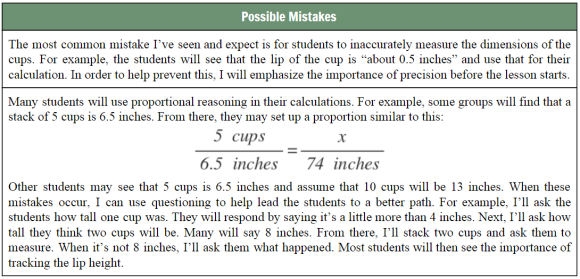
Monitoring

“One way to facilitate the monitoring process is for the teacher, before beginning the lesson, to create a list of solutions that he or she anticipates that students will produce and that will help in accomplishing his or her mathematical goals for the lesson.” (Smith & Stein, 2011)

After creating the list of possible solutions in the anticipation stage, I actively looked for these strategies during the group work session in order to take note of which groups were using what strategies. Here’s the monitoring chart I used.

As the groups worked, I noticed that most were using arithmetic in order to solve the problem. However, there was at least one group in each class that used an equation.

Selecting

“The selection of particular students and their solutions is guided by the mathematical goal for the lesson and the teacher’s assessment of how each contribution will contribute to that goal. Thus, the teacher selects certain students to present because of the mathematics in their responses.” (Smith & Stein, 2011)

Since most of the groups used the arithmetic method, I had one group present this method from each class. I also selected a group that used equations. Also, I chose speakers based on who has and hasn’t presented in the past. I want to make sure as many students as possible get a chance to be the “expert” throughout the year.

Sequencing

“Having selected particular students to present, the teacher can then make decisions regarding how to sequence the student presentations. By making purposeful choices about the order in which students’ work is shared, teachers can maximize the chances of achieving their mathematical goals for the discussion.” (Smith & Stein, 2011)

For the sequencing process, I decided to have a group present the most commonly used strategy first. This is a recommendation from the book that I like because it allows the highest percentage of students to have investment in the presentation process. Since most of the groups used the arithmetic method, they would be immediately curious to see how the first presentation compared to their work.

After a group presented the arithmetic method, I had a group that used equations present next. Specifically, I wanted to highlight their thought process for determining where to put the numbers in the equation. The intention was to lead the class closer to an understanding of slope and the y-intercept.

Connecting

“Rather than having mathematical discussions consist of separate presentations of different ways to solve a particular problem, the goal is to have student presentations build on one another to develop powerful mathematical ideas.” (Smith & Stein, 2011)

After the presentations were complete, I led the students through a connection handout. These handouts are my preferred way to ensure that the learning goal is met. The handout starts by highlighting key methods at the top. From there, leading questions are asked and big ideas for the unit (I stole them from New Visions for Public Schools) are mentioned in order to make connections and prepare students for developing procedural fluency in the coming days.

Materials:

Here are the links to all of the materials mentioned in this post and used during the lesson.

Smith, M., & Stein, M. (2011). 5 Practices for Orchestrating Productive Mathematics Discussions. Reston, VA: National Council of Teachers of Mathematics